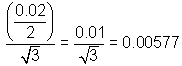SUPPLEMENTAL DOCUMENT SD-3
FOR PART IVC – Quality Assurance/Uncertainty
Measurement
Uncertainty for Weight Determinations in Seized Drug Analysis
NOTE: Changes have been
incorporated throughout Revision 2 to include a Table of Contents, an
additional example of Dynamic Weighing and a more thorough discussion of
correlation.
Table of
Contents
Introduction.. 3
A Example 1: Dynamic Weighing of a
Single Item Using a Budget Table. 3
A.1 Factors
contributing to weight measurement uncertainty. 4
A.2 Uncertainty
Budget Table. 5
A.3 Notes for the
Uncertainty Budget Table (A.2) 6
A.4 Calculation of
combined standard uncertainty............................................................ 7
A.5 Calculation of
expanded uncertainty. 7
A.6
Results. 8
B Example 2: Static Weighing of a
Single Item Using a Budget table. 9
B.1 Factors contributing to weight measurement
uncertainty. 9
B.2 Uncertainty Budget Table. 9
B.3 Calculation of combined standard uncertainty. 9
B.4 Calculation of expanded uncertainty. 10
B.5 Results. 10
C Example 3: Static Weighing of a Single Item Using Control
Chart Data in a Budget Table 11
C.1 Factors contributing to weight measurement
uncertainty. 11
C.2 Uncertainty Budget Table. 12
C.3 Notes for the Uncertainty Budget Table (C.2) 12
C.4 Calculation of combined standard uncertainty. 13
C.5 Calculation of expanded uncertainty. 13
C.6 Results. 13
D Example 4: Static Weighing of
Multiple Items to Obtain a Total Net Weight 14
D.1 Factors contributing to weight measurement uncertainty. 14
D.2 Uncertainty Budget Table. 14
D.3 Calculation of combined standard uncertainty. 14
D.4 Calculation of expanded uncertainty. 15
D.5 Results. 15
E References. 16
E.1 Books. 16
E.2 Journal articles and reviews. 16
E.3 On-line resources. 18
Introduction
The
following examples demonstrate the application of an uncertainty budget
approach for weight determinations and should be used in conjunction with
SWGDRUG Recommendations Part IV C, Section 4. These examples are designed to assist
laboratories in developing uncertainty budgets relevant to their procedures and
practices. They should not be directly
applied to methodology used in laboratories without first considering the specific
purpose of a method, its relevant operational environment and the operational
capabilities and parameters of the balance.
It is
assumed that the value being reported is the conventional mass and final
results are rounded to the precision of the balance. The calculations shown in the uncertainty
budgets were done using a spreadsheet. In some intermediate calculations,
additional digits are shown for illustrative purposes. How the results are reported would depend on
laboratory policy. The term “weight” is
used interchangeably with “conventional mass”, the quantity typically
reported. Definitions for the
statistical terms used can be found in the SWGDRUG glossary Part IV C, Annex A
or references listed below. The
references also contain additional examples and detailed information regarding
estimation of uncertainty.
Weighings
can be obtained using dynamic or static operations. A dynamic weighing process involves placing a
weighing vessel on a balance, taring the balance, and adding material immediately
to the weighing vessel without removing it from the balance. A static weighing process involves removal of
the tared weighing vessel, filling with material, and then returning to the
balance to obtain the net weight.
Examples are included in this document for both scenarios.
When
the determination of a net weight requires more than one weighing event, the
weighing events may be uncorrelated, correlated, or partially correlated. In practice the correlation, measured as the
correlation coefficient (r), is difficult to determine. In the following examples, values of r have
not been empirically determined. Rather, values of r have been selected to
represent a conservative approach which will likely result in an overestimation
of the measurement uncertainty. Where
applicable, references are provided for laboratories that elect to establish
their own correlation values.
A Example
1: Dynamic Weighing of a Single Item Using a Budget Table
Scenario: A laboratory must determine the
weight of a white powder (approximately 30 g) received in a plastic bag. The bag and its contents are considered to be
one item. The decision is made to weigh the material using a two place
(readability of 0.01g) balance. The
following conditions apply: the operator is competent on the use of the
balance; the balance is calibrated and certified as per established laboratory
protocols; the balance is being used above the defined minimum balance load;
and the balance is performing within the manufacturers’ specifications. The balance operates in a controlled
environment using a draft shield with ambient temperature varying within a
range of ±5 °C.
The
weight is determined as follows: A weighing vessel is placed on the balance and
tared. The analyst immediately transfers
the contents of the plastic bag to the tared weighing vessel without removing
it from the balance and records the net weight of the material. The entire operation is considered as a
single weighing event (a dynamic weighing).
The net
weight obtained for the powder is 30.03 grams.
A.1 Factors
contributing to weight measurement uncertainty
The factors
considered in estimating the measurement uncertainty include readability;
repeatability; linearity; buoyancy; temperature effects; uncertainty from the
balance calibration report; and sample loss in transfer. Although in some cases sample losses could be
large, the inability to accurately estimate the uncertainty due to sample
losses is not deemed a major concern since sample losses always result in
underestimation of the quantity of a substance being weighed. Therefore, uncertainty due to sample loss is
not included in any of the uncertainty computations given in this document (SD-3).
Buoyancy is difficult
to account for in seized drug cases because the density of the material being
weighed must be known. However, for
material that has a lower density than the steel calibration weights (8.0 g/cm3)
the bias imparted is always negative and the weight displayed by the balance
will be less than the true weight of the material. Ignoring buoyancy contributes a small
systematic error that represents no more than 0.1% bias to the weight. Therefore, buoyancy corrections are not made
in any uncertainty computations shown in this document (SD-3).
Based on the current
calibration and performance certification for the balance and given that the
balance is operating within specifications, other factors (e.g. environmental,
static electricity, corner loading) are deemed insignificant in this
example. Laboratories should examine
their balances, calibration reports, methods, circumstances, and applications to
determine which factors are significant and which are insignificant for their
particular application.
The factors deemed
significant in this example are expressed in the budget table to follow.
|
Factors
|
Valuea
|
Distribution
|
Standard uncertainty (u), g
|
Index
(Relative contribution)b
|
|
Readabilityc,d
|
0.01 g
|
Rectangular
|

|

|
|
Repeatability
(s)e
|
0.010 g
|
Normal
|
0.010
|
54.1%
|
|
Linearityc,f
|
0.02 g
|
Rectangular
|
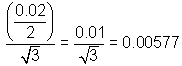
|
18.0%
|
|
Temperature
coefficientg
|
6 ppm/°C
( 6x10-6g/g /°C)
|
Rectangular
|

|
0.1%
|
|
Uncertainty
from balance calibration report (U,
coverage factor k=2)h
|
0.0131 g
|
Normal
|

|
23.2%
|
|
Subtotal of individual u
values:
|
(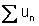 ): ):
|
0.02573
|
Sum of relative contributions: 100%
|
|
Subtotal of squared u values:
|
(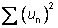 ): ):
|
0.0001848
|
|
a. This
value could be a standard uncertainty, the range or half-width of a rectangular
distribution, an expanded uncertainty, or some other quantity reported to
express the level of uncertainty arising from a specified source. The value of all factors should be in the
same units.
b.  This
value is used to determine which factors are significant.
This
value is used to determine which factors are significant.
c.
The
range of a rectangular distribution is from -a to +a. Therefore, half the value of the full range
is used to determine the standard uncertainty.
d. Obtained
from the current calibration and performance certification for the balance and
assumes that the balance has a single readability range.
e.
Determined empirically in the laboratory.
f. This value is the maximum permitted
deviation across the mass range of the balance.
g. Value obtained from manufacturer
specifications is ± 5°C. Since the
distribution is rectangular, half the range is used (5°C).
h. A conservative approach would involve the
measurement of uncertainty of the balance calibration at the upper working mass
range of the balance.
Considering all
factors noted above (A.2) as uncorrelated for a single weighing event, the
combined standard uncertainty can be expressed mathematically as:
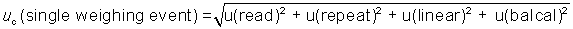
where u is the standard uncertainty and uc is combined standard
uncertainty. The factor u(temperature coefficient) is not
included in the combined uncertainty due to its minimal relative contribution
to the total standard uncertainty.
Therefore, the
combined standard uncertainty is:

Uc = 0.0136
The expanded
uncertainty is expressed mathematically as:
U = k*uc
Using a coverage
factor k = 2 (confidence level of
approximately 95%, assuming the net mass follows a normal distribution[I]):
U = 2*0.0136 g = 0.0272
g
Using a coverage
factor k = 3 (confidence level of
approximately 99% assuming the net mass follows a normal distribution):
U = 3*0.0136 g = 0.0408 g
A.6.1 Net Weight: 30.03 g ± 0.03 g (k=2)
A.6.2 Net Weight: 30.03 g ± 0.04 g (k=3)
B Example
2: Static Weighing of a Single Item Using a Budget Table
Scenario: The scenario is the same as in Example
1.
The
weight is determined as follows: A
weighing vessel is placed on the balance and tared. It is then removed from the balance and the
powder is transferred to the weighing vessel, which is placed on the balance
and a reading obtained (a static weighing).
The net
weight obtained for the powder is 30.03 grams.
The factors are the
same as in Example 1.
The uncertainty
budget table is the same as in Example 1. The factor u(temperature coefficient) is not included in the combined
uncertainty due to its minimal relative contribution to the total standard
uncertainty.
The combined standard
uncertainty for a single weighing event is the same as in Example 1:

uc = 0.0136
In this case, the calculation of total
uncertainty for the net mass is:
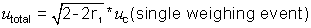
where r1
is the correlation coefficient between the weighing associated with the tare
and the weighing of the material.
Because this is a static weighing process, two separate weighing events
are considered-the taring of the weigh vessel and the weighing of the material.
In practice, the
value of r1 is difficult to determine.
In this example, the most conservative approach is to assume that the two
weighing events are completely negatively correlated. As a result, r1 is assigned the
value of -1. This will likely result in overestimation of the uncertainty.

utotal = 2 * 0.0136 g =
0.0272 g
Alternatively, the
laboratory may elect to empirically determine another value for r1.
The expanded
uncertainty is expressed mathematically as:
U = k*utotal
Using a coverage
factor k = 2 (confidence level of
approximately 95%, assuming the net mass follows a normal distribution):
U = 2*0.0272 g = 0.0544
g
Using a coverage
factor k = 3 (confidence level of
approximately 99% assuming the net mass follows a normal
distribution):
U = 3*0.0272 g = 0.0816 g
B.5.1 Net Weight: 30.03 g ± 0.05 g (k=2)
B.5.2 Net Weight: 30.03 g ± 0.08 g (k=3)
C Example 3: Static Weighing of a Single
Item Using Control Chart Data in a
Budget Table
Scenario: In this example, the measurement
uncertainty is calculated using control chart data obtained from a measurement
quality assurance process that mimics casework samples as closely as possible.
All other conditions and assumptions are the same as Example 2, including the
use of a static weighing process.
The
control chart should capture uncertainty deemed appropriate to the specific
laboratory and procedure and could include factors such as environmental
conditions, analysts, and sample types.
A conservative approach is to select the largest standard deviation if a
range of masses is charted.
As the laboratory’s
control chart is well established, it is expected to capture all of the factors
described in Example 1 except linearity and balance calibration uncertainty.
|
Factors
|
Value
|
Distribution
|
Standard uncertainty (u), g
|
Index
(Relative contribution)
|
|
Control
chart standard deviation (s)a
|
0.0313 g
|
Normal
|
0.0313
|
92.8 %
|
|
Linearityb
|
0.02 g
|
Rectangular
|
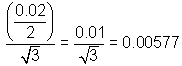
|
3.2 %
|
|
Uncertainty
from balance calibration report (U,
coverage factor k=2)
|
0.0131 g
|
Normal
|

|
4.1 %
|
|
Subtotal of individual u
values:
|
( ): ):
|
0.0436
|
Sum of
relative contributions: 100%
|
|
Subtotal of squared u values:
|
(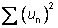 ): ):
|
0.00106
|
|
a. 

b. This value is the maximum permitted deviation
across the mass range of the balance.
The combined standard
uncertainty per weighing event can be expressed in this example mathematically
as:

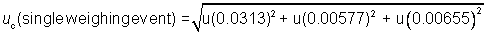
Under the assumption
about correlation between the sample and tare weighings made in Example 2, the
total uncertainty is:
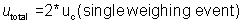
utotal = 2 * 0.0325 g =
0.0650 g
The expanded
uncertainty is expressed mathematically as:
U = k*utotal
Using a coverage
factor k = 2 (confidence level of
approximately 95% assuming the net mass follows a normal distribution):
U = 2*0.0650 g = 0.130
g
Using a coverage
factor k = 3 (confidence level of
approximately 99% assuming the net mass follows a normal distribution 2):
U = 3*0.0650 g = 0.195 g
C.6.1 Net Weight: 30.03 g ± 0.13 g (k=2)
C.6.2 Net Weight: 30.03 g ± 0.20 g (k=3)
D Example 4: Static Weighing of Multiple
Items to Obtain a Total Net Weight
Scenario: In this example, the laboratory must
determine the net weight of a white powder, received in 15 similar plastic bags
(15 items), which appear to weigh approximately 30 g each. All other conditions are the same as Example
3.
The net
weight of the contents of each bag was determined as in Examples 2 and 3 (a
static weighing procedure). The total net weight obtained for the powder,
determined by individually placing the material from each plastic bag inside 15
separate tared weighing vessels, is 458.37 grams.
Same as in Example
3. The laboratory could also choose to
use the uncertainty budget approach as presented in Example 2.
Same
as Example 3. The laboratory could also choose to use the
uncertainty budget as presented in Example 2.
Using the correlation
between weighing of sample and tare assumed in Examples 2 and 3, the
calculation of total uncertainty for the net mass of the material from all 15
bags is:
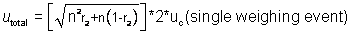
where r2
is a correlation coefficient describing the relationship between the weighings
of any pair of bags. Note this
correlation (r2) is not the same correlation as between the tare and
the weighing (r1).
In practice, the
value of r2 is difficult to determine.
In this example, the most conservative approach is to assume that the weighings
between any two bags are all completely positively correlated. As a result, r2 is assigned the
value of +1. This will likely result in
overestimation of the uncertainty.

utotal
= 15 items * 2 * 0.0325 g = 0.975 g
Alternatively, the
laboratory may elect to empirically determine another value for r2.
The expanded
uncertainty per weighing event (U) is
expressed mathematically as:
U = k*utotal
Using a coverage
factor k = 2 (confidence level of
approximately 95%):
U = 2*0.975 g = 1.95 g
Using a coverage
factor k = 3 (confidence level of
approximately 99%):
U = 3*0.975 g = 2.93 g
D.5.1 Net Weight: 458.37 g ± 1.95 g (k=2)
D.5.2 Net Weight: 458.37 g ± 2.93 g
(k=3)
E References
E.1.1
Dieck,
R.H. 2007. Measurement Uncertainty: Methods and Applications. 4th ed. Research Triangle Park, NC: The Instruments, Systems, and Automation
Society (ISA).
E.1.2
Jones,
F. E., and R. M. Schoonover. 2002. Handbook
of Mass Measurement. Boca Raton,
FL: CRC Press.
E.1.3
Kimothi, S.K. 2002. The Uncertainty of Measurements: Physical and Chemical Metrology:
Impact and Analysis. Milwaukee,
WI: ASQ Press (American Society
for Quality).
E.1.4
Kirkup,
L., and R. B. Frenkel. 2006. An
Introduction to Uncertainty in Measurement. Cambridge,
UK: Cambridge University
Press.
E.1.5
Lawn,
R., and E. Pritchard. 2003. Measurement
of Mass, Practical Laboratory Skills Training Guides Series: Royal Society
of Chemistry.
E.1.6
Pavese,
F., and A.B. Forbes, eds. 2009. Data
Modeling for Metrology and Testing in Measurement Science. Edited by N. Bellomo, Modeling and Simulation in Science,
Engineering, and Technology. Boston:
Birkhauser.
E.1.7
Taylor,
J.R. 1997. An Introduction to Error
Analysis: The Study of Uncertainties in Physical Measurements (2nd ed).
Sausalito, CA: University Science Books.
E.2.1
Bremser,
W., J. Viallon, and R. I. Wielgosz. 2006. Influence of correlation on the
assessment of measurement result compatibility over a dynamic range. Metrologia 44(6): 495-504.
E.2.2
Brown,
R. J. C., and M. J. T. Milton. 2007. Developments in accurate and traceable
chemical measurements. Chemical Society
Reviews 36 (6):904-913.
E.2.3
Burns,
M. 2004. Current practice in the assessment and control of measurement
uncertainty in bio-analytical chemistry. Trac-Trends
in Analytical Chemistry 23(5):393-398.
E.2.4
Choi,
J., E. Hwang, H. Y. So, and B. Kim. 2003. An uncertainty evaluation for multiple
measurements by GUM. Accreditation and
Quality Assurance 8(1): 13-15.
E.2.5
Ellison,
S. L. R. 2005. Including correlation effects in an improved spreadsheet
calculation of combined standard uncertainties. Accreditation and Quality Assurance 10(7):338-43.
E.2.6
Gonzalez, A. G., and M. A. Herrador. 2007.
The assessment of electronic balances for accuracy of mass measurements in the
analytical laboratory. Accreditation and
Quality Assurance 12(1):21-29.
E.2.7
Haesselbarth,
W., and W. Bremser. 2004. Correlation between Repeated Measurements: Bane and
Boon of the GUM Approach to the Uncertainty of Measurement. Accreditation and Quality Assurance 9(10):
597-600.
E.2.8
Hasselbarth,
W., and W. Bremser. 2007. Measurement uncertainty for multiple measurands:
characterization and comparison of uncertainty matrices. Metrologia 44(2):128-45.
E.2.9
Kehl, K. G., K.
Weirauch, S. Wunderli, and V. R. Meyer. 2000. The influence of variations in
atmospheric pressure on the uncertainty budget of weighing results. Analyst 125(5):959-962.
E.2.10 Kessel, R., M. Berglund, P. Taylor, and R
Wellum. 2001. How to treat correlation in the uncertainty budget, when combining
results from different measurements, in Advanced
Mathematical and Computational Tools in Metrology V, edited by P. Ciarlini,
M.G. Cox, E. Filipe, F. Paveses and D. Richter. Series on Advances in
Mathematics for Applied Sciences - Vol. 57. Singapore: World Scientific
Publishing, 2001, p. 231-241.
E.2.11 Kessel, R., R. Kacker,
and M. Berglund. 2006.
Coefficient of contribution to the combined standard uncertainty. Metrologia 43(4): S189-S95.
E.2.12 Konieczka, P. 2007. The role of and the place
of method validation in the quality assurance and quality control (QA/QC)
system. Critical Reviews in Analytical
Chemistry 37(3):173-190.
E.2.13 Linsinger, T. P. J. 2008. Use of recovery and
bias information in analytical chemistry and estimation of its uncertainty
contribution. Trac-Trends in Analytical Chemistry 27 (10):916-923.
E.2.14 Meyer, V. R. 2007. Measurement uncertainty. Journal of Chromatography A 1158
(1-2):15-24.
E.2.15 Populaire, S., and E. C. Gimenez. 2006. A
simplified approach to the estimation of analytical measurement uncertainty. Accreditation and Quality Assurance 10
(9):485-493.
E.2.16 Pozivil, M., W. Winiger, S.
Wunderli, and V. R. Meyer. 2006. The influence of climate conditions on weighing
results. Microchimica Acta 154
(1-2):55-63.
E.2.17 Rios, A., and M. Valcarcel. 1998. A view of
uncertainty at the bench analytical level. Accreditation
and Quality Assurance 3 (1):14-19.
E.2.18 Schoonover, R. M., and F. E. Jones. 1981. Air
Buoyancy Correction in High-Accuracy Weighing on Analytical Balances. Analytical Chemistry 53 (6):900-902.
E.2.19 Wunderli, S., G. Fortunato, A. Reichmuth, and
P. Richard. 2003. Uncertainty evaluation of mass values determined by
electronic balances in analytical chemistry: a new method to correct for air
buoyancy. Analytical and Bioanalytical
Chemistry 376 (3):384-391.
E.3.1
EURACHEM.
Quantifying Uncertainty in Analytical Measurement 2007. Available from http://www.measurementuncertainty.org/index.html.
E.3.2
JCGM.
JCGM 100-2008: Evaluation of measurement data (GUM 1995 with minor
corrections). JCGM: Joint Committee for Guides in Metrology, 2008 2008.
Available from http://www.iso.org/sites/JCGM/GUM/JCGM100/C045315e-html/C045315e.html?csnumber=50461.
E.3.3
NIST.
2006. NIST/SEMATECH e-Handbook of Statistical Methods: National Institute of
Science and Technology, U.S. Department of Commerce. Available
from http://www.itl.nist.gov/div898/handbook.
E.3.4
NIST.
2000. The NIST Reference on Constants, Units, and Uncertainty. Available from http://physics.nist.gov/cuu/Uncertainty/index.html.
E.3.5
NIST
1994. NIST Technical note 1297 Guidelines for Evaluating and Expressing the
Uncertainty of NIST Measurement Results. National Institute
of Science and Technology, U.S.
Department of Commerce. Available from http://physics.nist.gov/Pubs/guidelines/contents.html.
E.3.6
SIM
Guidelines on the calibration of non-automatic weighing instruments. 2009. SIM MWG7/cg-01/v.00. (Identical to the
original EURAMET/cg-18/v.02) Available from http://www.dkd.eu/dokumente/Richtlinien/EURAMET_cg-18_v.02.pdf.
End of
Document
The approximate confidence levels
given in this document (SD-3) assume that the quantities for which expanded
uncertainties are being computed each approximately follow a normal
distribution. If this assumption does
not hold, the actual confidence level attained for these uncertainty intervals
may be lower or higher than the desired levels of 95% or 99%
If not known from relevant references in the metrology
literature or experience, the value of the correlation coefficient r1
may be determined empirically by performing an experiment with mass standards
that approximate the gross and tare masses of the seized drugs on the
balance. If the correlation between the
gross and tare masses is based on common corrections applied in the computation
of each mass, the value of the correlation coefficient r1 can be
obtained using the methods in Section F.1.2.3 of the GUM[D.3.2]. Several articles addressing correlation are
included in Section E.2.
If not known from
relevant references in the metrology literature or experience, the value of the
correlation coefficient r2 may be determined empirically by
performing an experiment with measurement assurance standards that approximate
composition and masses of the bags in the case. If the correlation between
masses of different bags is based on common corrections applied in the
computation of each mass, the value of the correlation coefficient r2
can be obtained using the methods in Section F.1.2.3 of the GUM [E.3.2]. Several
articles discussing correlation are included in Section E.2.